Chair of Production Management, University of Regensburg
Dissertation Project
Analysis of buffer allocations in time-dependent and stochastic flow lines
Date of Defense
11 December 2015
Summary
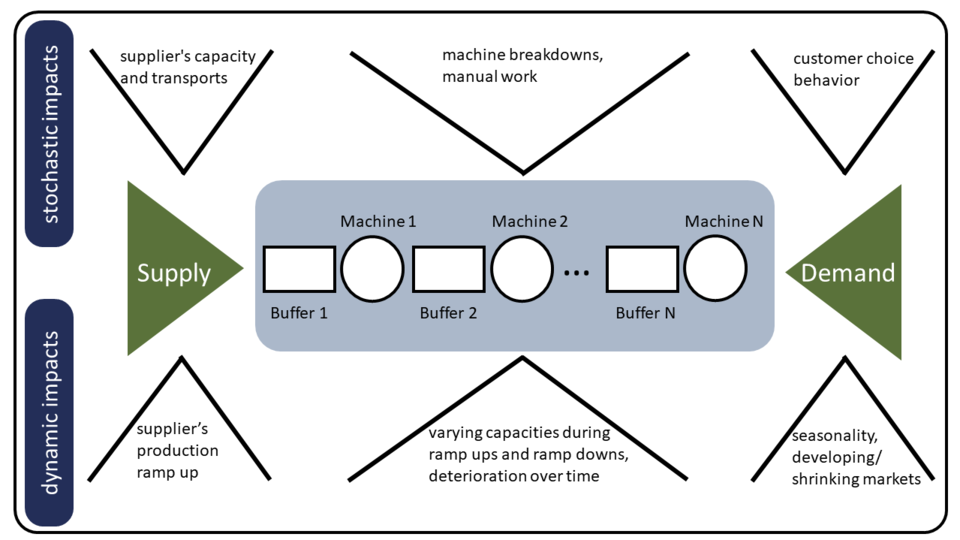
Flow lines are production systems that can be found, e.g., in the food and automotive industries, for production at high volume. Modern information technology allows flexible and short-term changes of buffer capacities in flow lines by means of control mechanisms such as electronic Kanban cards. This thesis investigates the potential of time-dependent adjustments of buffer capacities to account for time-dependent changes in demand and machine characteristics. Performance evaluation approaches for given buffer allocations and algorithms to systematically derive time-dependent buffer allocations are developed. In contrast, the existing literature typically assumes constant buffer capacities and treats their allocation as a long-term design problem. The first essay presents an overview of the existing literature on buffer capacity optimization in unreliable flow lines. All reviewed articles assume that flow lines operate under steady-state conditions. The second essay provides a survey and classification of performance evaluation approaches for queueing systems with time-dependent parameters and their applications. It is apparent that, even for single-stage systems with finite buffer capacities, there are no exact analytical solutions. The third essay introduces two sample-based approaches for the performance evaluation of flow lines with a time-dependent processing rate on the first machine and constant buffer capacities and constant rates for the subsequent machines. The equivalence of the two approaches has been established for a special case. The numerical study demonstrates that, given a time-dependent input, buffers can smooth the output over time. The fourth essay proposes a sample-based evaluation approach that accounts for time-dependent buffer capacities. The numerical study indicates
the potential for influencing key performance measurements, such as work in process inventory (WIP) and throughput by time-dependent buffer allocations. The fifth essay reports monotonicity properties for the WIP and the service level of a flow line with respect to time-dependent buffer capacities based on a numerical study. A search algorithm utilizes these properties to find time-dependent buffer capacities. The numerical study indicates that the generated solutions lead to lower average WIP compared to constant buffer capacities while satisfying the same service level goal.